L.  Richter¹, E. Brehm² and C.-A. Graubner³

1. Bilfinger Berger Nigeria GmbH, Wiesbaden, Germany, lars.richter@bilfinger.de
2. D. candidate, Institute of Concrete Structures and Materials, Technische Universität Darmstadt, Darmstadt, Germany, brehm@massivbau.tu-darmstadt.de
3. Professor, Institute of Concrete Structures and Materials, Technische Universität Darmstadt, Darmstadt, Germany, graubner@massivbau.tu-darmstadt.de

ABSTRACT

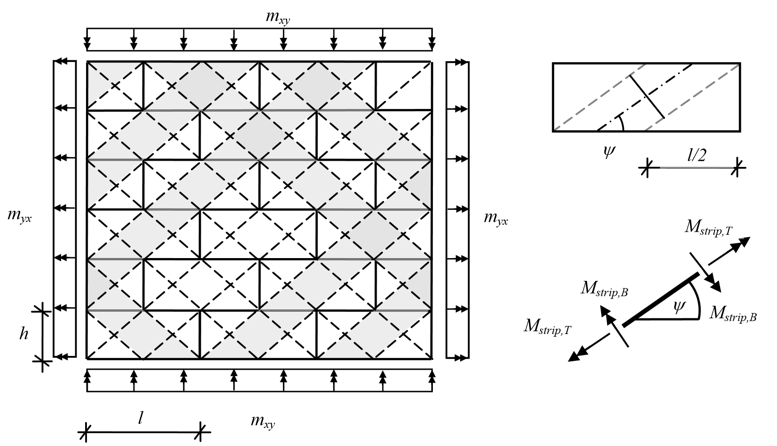
The design of unreinforced masonry walls subjected to uniformly distributed lateral loading and simply supported on more than two edges is based on the yield line method or a modification thereof (e.g. British Standard, European Standard, Canadian Standard). In the yield line method, plastic behaviour of the material is assumed as shown by steel and reinforced concrete. The analysis of the load-bearing behaviour of masonry has to be divided into two directions. Normal to the bed joint, the plastic behaviour depends on the ratio of the axial load to the corresponding flexural tensile strength of masonry. The transfer of horizontal bending moment depends on the available flexural strength of the units and torsional shear strength of the bed joints. Therefore, two different failure modes with unequal plastic behaviour have to be considered. On the one hand, the unit can fail due to flexural tensile stress (brittle failure), and no residual load-bearing capacity after cracking exists. On the other hand, failure can occur due to the torsional failure in the bed joints, and a plastic moment can transfer depending on the level of normal stresses in the bed joints. The complex load-bearing behaviour of masonry shows that the analysis of flexural wall panels using the yield line theory depends on the axial load and the material strength of the units and mortar, as well as their bond. To consider these different residual load–bearing capacities, a new non-linear method of analysis was developed. This paper will present a simplified design model for non-load bearing walls based on the new calculation method.

KEYWORDS: masonry, load bearing capacity, flexural tensile strength, bending, biaxial

C1-2